Figure out the Number Before Percentage Increase
Introducing our online calculator, designed to swiftly calculate the number before a percentage increase with ease and precision. Whether you're analyzing finances or solving math problems, our intuitive interface simplifies the process. Say goodbye to manual calculations and streamline your work with our efficient online percentage calculator.
Quick tip:
Press Tab key to move to the next field. Press Shift + Tab keys to move to the previous field. Press Enter key to calculate the result.
Calculate number before percentage increase
final
inc.%
How to calculate the number before a percentage increase
To find the original number before a percentage increase, you can reverse the calculation. Here's a step-by-step guide on how to do it.
Step 1: Understand the Percentage Increase
Percentage increase tells you how much the original number has been increased by a certain percentage. For example, if a number increases by 20%, you know that the final number is 120% of the original.
Step 2: Gather Your Numbers
You need two numbers: the final number (after the increase) and the percentage of increase. For example:
- After: 120 (the number after the increase)
- Percentage Increase: 20%
Step 3: Convert the Percentage Increase to a Decimal
Add the percentage increase to 100 to get the percentage of the original number. Then convert that percentage into a decimal. For example:
Percentage after increase=100% + 20%=120%
As a decimal: 120%=1.20
Step 4: Calculate the Original Number
To find the original number, divide the final number by the decimal from Step 3:
Original Number=Final Number / Decimal
For this example: Original Number=120 / 1.20=100
Step 5: Verify Your Result
You can verify the calculation by checking if the original number plus the percentage increase gives you the final number:
Original Number + (20% of Original Number)=Final Number
100 + (20% of 100)=120
Let's Recap:
- Understand the Percentage Increase: Know how much the number increased.
- Gather Your Numbers: After the increase and the percentage increase.
- Convert to Decimal: Add the percentage increase to 100 and convert to a decimal.
- Calculate the Original Number: Divide the final number by the decimal.
- Verify the Result: Check if adding the percentage increase to the original gives the final number.
Calculate number before percentage increase in Excel or Google Sheets
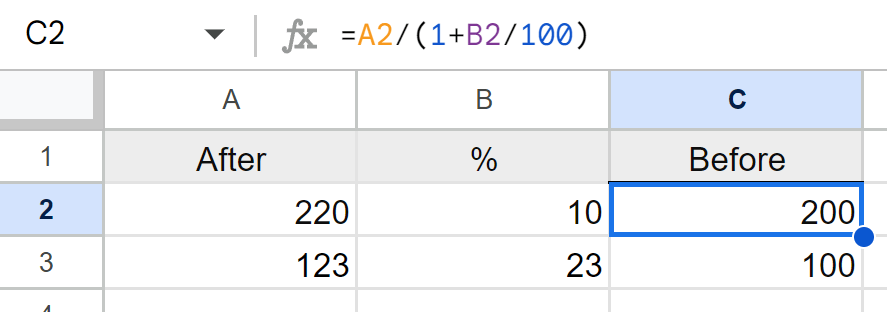
Formula is =A2/(1+B2/100), where A2 is the "After" number, and B2 is the "Percentage".
- After: This column represents the number after the percentage increase.
- Before: This column represents the number before the percentage increase.
- %: This column displays the percentage by which the initial number was increased.
Popular Calculations to Find the Number Before Percentage Increase
1. Original Price Before Markup
Scenario: A product costs $120 after a 20% markup. What was the original price?
Calculation: Find the original price before a 20% increase.
Let X be the original price. The final price is 120% of the original price.
$120=X × (1 + 0.20)
$120=X × 1.20
X=$120 / 1.20=$100
Result: The original price before the 20% markup was $100.
2. Salary Before Increase
Scenario: Your salary is now $55,000 after a 10% increase. What was your salary before the increase?
Calculation: Find the salary before a 10% increase.
Let X be the original salary. The current salary is 110% of the original salary.
$55,000=X × (1 + 0.10)
$55,000=X × 1.10
X=$55,000 / 1.10=$50,000
Result: The salary before the 10% increase was $50,000.
3. Cost Before Tax
Scenario: A meal costs $138 after including an 8% tax. What was the cost before tax?
Calculation: Find the cost before an 8% increase.
Let X be the cost before tax. The final cost is 108% of the pre-tax cost.
$138=X × (1 + 0.08)
$138=X × 1.08
X=$138 / 1.08=$127.78
Result: The cost before tax was approximately $127.78.
4. Tuition Fee Before Increase
Scenario: A tuition fee of $1,200 is the result after a 15% increase. What was the original fee?
Calculation: Find the original fee before a 15% increase.
Let X be the original fee. The final fee is 115% of the original fee.
$1,200=X × (1 + 0.15)
$1,200=X × 1.15
X=$1,200 / 1.15=$1,043.48
Result: The original tuition fee was approximately $1,043.48.
5. Value Before Appreciation
Scenario: An asset is worth $900 after a 25% appreciation. What was its original value?
Calculation: Find the value before a 25% increase.
Let X be the original value. The appreciated value is 125% of the original value.
$900=X × (1 + 0.25)
$900=X × 1.25
X=$900 / 1.25=$720
Result: The value before the 25% appreciation was $720.